Flow past a circular cylinder: Numerical convergence and computational efficiency
Hongyi Jiang (University of Western Australia)
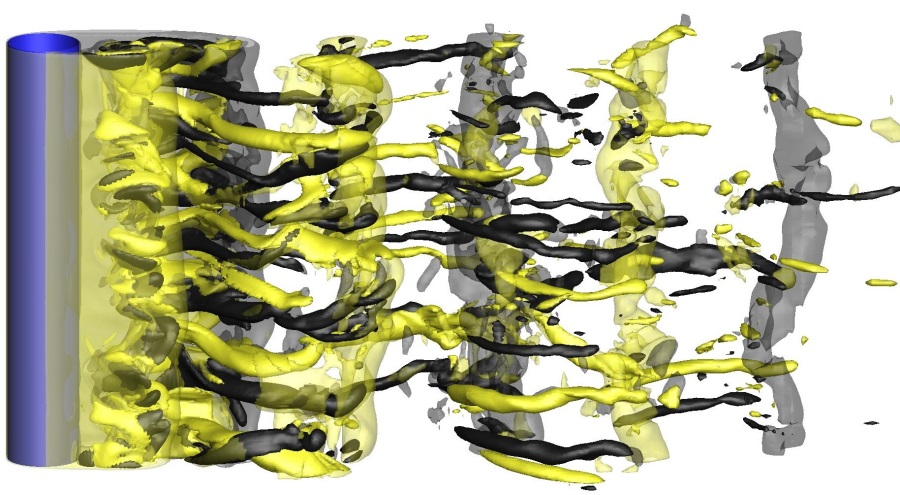
The case of a steady approaching flow past a slender and smooth circular cylinder has been a classical problem in fluid mechanics for over one hundred years, owing to its fundamental significance and extensive practical applications. After presenting an overview of my numerical investigations on the flow past a circular cylinder over the past few years, this presentation will focus mainly on the numerical convergence and computational efficiency for a popular benchmarking case at a Reynolds number (Re) of 3900. The case Re = 3900 was computed with two open-source codes, Nektar++ and OpenFOAM. By using Nektar++, mesh convergence for the case Re = 3900 was demonstrated through a systematic mesh dependence study. The same case was also simulated with OpenFOAM (v5.0), and it was found that the Nektar++ approach was more computationally efficient. Based on the Nektar++ approach, the computations were then generalized to a range of Re, and the high-fidelity data were used to analyse the flow physics.